

Refractometry:
Theory
The speed of light in a vacuum is always the same, but when light moves through any other medium it travels more slowly since it is constantly being absorbed and reemitted by the atoms in the material. The ratio of the speed of light in a vacuum to the speed of light in another substance is defined as the index of refraction (aka refractive index or n) for the substance.
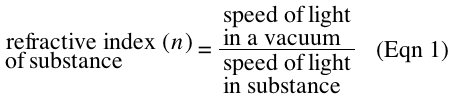
 |
Figure 1. Light crossing from any transparent medium into another in which it has a different speed, is refracted, i.e., bent from its original path (except when the direction of travel is perpendicular to the boundary). In the case shown, the speed of light in medium A is greater than the speed of light in medium B. |
Whenever light changes speed as it crosses a boundary from one medium into another its direction of travel also changes, i.e., it is refracted (Figure 1). (In the special case of the light traveling perpendicular to the boundary there is no change in direction upon entering the new medium.) The relationship between light's speed in the two mediums (vA and vB), the angles of incidence (qA) and refraction (qB) and the refractive indexes of the two mediums (nA and nB) is shown below:
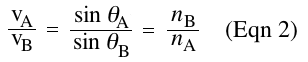
Thus, it is not necessary to measure the speed of light in a sample in order to determine its index of refraction. Instead, by measuring the angle of refraction, and knowing the index of refraction of the layer that is in contact with the sample, it is possible to determine the refractive index of the sample quite accurately. Nearly all refractometers utilize this principle, but may differ in their optical design.
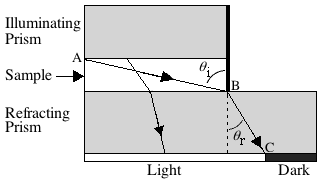 |
Figure 2. Cross section of part of the optical path of an Abbe refractometer. The sample thickness has been exaggerated for clarity. |
In the Abbe' refractometer the liquid sample is sandwiched into a thin layer between an illuminating prism and a refracting prism (Figure 2). The refracting prism is made of a glass with a high refractive index (e.g., 1.75) and the refractometer is designed to be used with samples having a refractive index smaller than that of the refracting prism. A light source is projected through the illuminating prism, the bottom surface of which is ground (i.e., roughened like a ground-glass joint), so each point on this surface can be thought of as generating light rays traveling in all directions. Inspection of Figure 2 shows that light traveling from point A to point B will have the largest angle of incidence (qi) and hence the largest possible angle of refraction (qr) for that sample. All other rays of light entering the refracting prism will have smaller qr and hence lie to the left of point C. Thus, a detector placed on the back side of the refracting prism would show a light region to the left and a dark region to the right.
Samples with different refractive indexes will produce different angles of refraction (see Equation 2 above and recall that the angle of incidence and the refractive index of the prism are fixed) and this will be reflected in a change in the position of the borderline between the light and dark regions. By appropriately calibrating the scale, the position of the borderline can be used to determine the refractive index of any sample. In an actual Abbe' refractometer there is not a detector on the back of the refracting prism, and there are additional optics, but this is the essential principle.
(It is also possible to design a refractometer based on the reflection of light from the boundary between the prism and the sample. These types of refractometers are often used for continuous monitoring of industrial processes.)
In most liquids and solids the speed of light, and hence the index of refraction, varies significantly with wavelength. (This variation is referred to as dispersion, and it is what causes white light moving through a prism to be refracted into a rainbow. Shorter wavelengths are normally refracted more than longer ones.) Thus, for the most accurate measurements it is necessary to use monochromatic light. The most widely used wavelength of light for refractometry is the sodium D line at 589 nm.
If white light were used in the simple Abbe' refractometer optics shown in Figure 2, dispersion would result in the light and dark borderline being in different places for different wavelengths of light. The resulting "fuzziness" of the borderline would make precise work impossible. However, many Abbe' refractometers are able to operate satisfactorily with white light by introducing a set of "compensating prisms" into the optical path after the refracting prism. These compensating prisms are designed so that they can be adjusted to correct (i.e., compensate for) the dispersion of the sample in such a way that they reproduce the refractive index that would be obtained with monochromatic light of 589 nm, the sodium D line.
As mentioned earlier, the speed of light in a substance is slower than in a vacuum since the light is being absorbed and reemitted by the atoms in the sample. Since the density of a liquid usually decreases with temperature, it is not surprising that the speed of light in a liquid will normally increase as the temperature increases. Thus, the index of refraction normally decreases as the temperature increases for a liquid (Table 1). For many organic liquids the index of refraction decreases by approximately 0.0005 for every 1 °C increase in temperature. However for water the variation is only about -0.0001/°C.
Many refractometers are equipped with a thermometer and a means of circulating water through the refractometer to maintain a given temperature. Most of the refractive index measurements reported in the literature are determined at 20 or 25 °C.
